“If, in some cataclysm, all of scientific knowledge were to be destroyed, and only one sentence passed on to the next generations of creatures, what statement would contain the most information in the fewest words? I believe it is the atomic hypothesis that all things are made of atoms” Richard Feynman
Jon Osler is a chemistry graduate of the University of St Andrews. He gained his PhD in chemistry from the University of York for his work on the Cope rearrangement with Professor Richard Taylor. Jon’s research has been published in a number of leading international journals. He is currently living in Paris, teaching chemistry for the International Baccalaureate Diploma at the Ecole Jeannine Manuel.
The ancient Greek philosopher, Democritus theorised that if one continued to cut matter in two, we would eventually arrive at some indivisible building block of matter, which he called atomos. However, it wasn’t until the industrial revolution that a model of the particulate nature of matter would be used to predict physical phenomena. The steam engine was changing the world and in order to understand how it worked we needed to understand and predict the behaviour of steam at high temperatures and pressures. Ludwig Boltzmann imagined steam as being made up of tiny spheres of matter in constant, rapid and random motion colliding with each other and the walls of any container. He created mathematical models based on these assumptions which could accurately predict the behaviour of steam. However, his models were controversial. His opponents argued that we could not see these particles and as such they were simply mathematical conveniences. So how do we know atoms exist? This is a complex question. We cannot see an atom with our own eyes as they are extremely small. The diameter of an atom ranges from about 1 x 10-10 m to 5 x 10-10 m, about a million times smaller than the thickest human hair. However, scientists are convinced of their existence and of their importance in our understanding of the universe. When asked “If, in some cataclysm, all of scientific knowledge were to be destroyed, and only one sentence passed on to the next generations of creatures, what statement would contain the most information in the fewest words?” the great physicist Richard Feynman replied “the atomic hypothesis that all things are made of atoms”. We know about these atoms because scientists have made observations, measurements and done a lot of thinking. Crucially, scientists are open to the possibility that they may be wrong and a framework of knowledge grows over time. Carl Sagan noted that “In science it often happens that scientists say, ‘You know that’s a really good argument; my position is mistaken,’ and then they would actually change their minds and you never hear that old view from them again. They really do it. It doesn’t happen as often as it should, because scientists are human and change is sometimes painful. But it happens every day. I cannot recall the last time something like that happened in politics or religion.” The story of how science has led us to the currently accepted structure of the atom shows how scientific facts, more accurately described as models which describe our universe, develop over time in the face of new evidence.
Brownian Motion
In 1905, Einstein lent his weight to the debate between Boltzmann and the non-atomists. Almost 80 years earlier, in 1827, a Scottish botanist called Robert Brown looked down a microscope at pollen grains floating on water. Surprisingly, he observed the pollen grains jiggling around in all sorts of directions as if they were alive. The erratic movements of these pollen grains is now called “Brownian motion”. Einstein realised its importance and that the simple observation of pollen jiggling about on the surface of water constituted evidence in support of the existence of atoms. Einstein’s reasoning was simple. The movement of the pollen grains on the surface of the water, makes it evident that the pollen grains are being jostled. The water must therefore be made of tiny particles which are themselves jiggling and jostling the pollen. Einstein showed that for Brownian motion to happen, tiny particles, so small that we can’t see them, must exist.
A video showing the Brownian motion of pollen grains floating on water
J.J. Thomson’s cathode rays
Democritus described his atomos as an indivisible particle. This is not how we see the atom today. The first indication that atoms may have an internal structure was an experiment carried out by J.J. Thomson, who was investigating how an electric current flows through a gas. Thomson fitted a glass tube with two electrodes. When the electrodes were connected to a power supply, coloured rays started streaming out of the cathode (the negatively charged electrode). Thomson could deflect these cathode rays by applying an external magnetic or electric field. The cathode rays were deflected towards a positive plate and repelled by a negative plate.
Thomson was able to infer much information from his observations. A series of complex calculations revealed that the cathode rays were moving slower than the speed of light and he reasoned that they were likely to be made of tiny particles rather than being a form of radiation. He deduced that because the rays came from the negative electrode and were deflected towards the positively charged plate, the particles that made up the cathode rays must be negatively charged. Because the rays were easily deflected by the magnetic and electric fields, he realised that the particles were very light. The fact that the beam did not broaden when deflected by the electric or magnetic field led him to conclude that the rays must have been made up of identical particles (with the same mass and charge) all moving at the same speed. If the rays had been made up of particles of different masses, the lighter particles would have been deflected more, and if they had been made up of particles with different charges, the more highly charged particles would have been deflected more. By fitting a hole in the positive electrode and a fluorescent screen beyond it, he was able to quantitatively measure the extent to which electric and magnetic fields deflected the cathode ray and calculate the charge to mass ratio of the tiny particle we now call the electron as 1.76 x 108 C g-1.
 Deflection of cathode rays by an electric field demonstrating that they are negatively charged (creative commons) Source: chemlibretexts.org
Deflection of cathode rays by an electric field demonstrating that they are negatively charged (creative commons) Source: chemlibretexts.org
Millikan’s oil drop experiment
Robert Millikan was able to determine the charge on the electron as 1.602 x 10-19 C by observing the rate of fall of charged oil droplets. Small drops of oil, which had picked up electrons were allowed to fall under gravity. However, a positively charged metal plate which had been set-up above the oil drops and a negatively charged plate below slowed their fall. By varying the potential difference (the difference in electric potential) across the plates and observing the effect this had on the rate of fall, he was able to calculate the charge on each droplet. All the values were found to be whole number multiples of 1.602 x 10-19 C depending on how many electrons had added to the oil droplet. Since Thomson had already identified the charge to mass ratio for the electron, knowing its charge enabled the mass of the electron to be determined as just 9.10 x 10-28 g.
Rutherford’s experiment on the scattering of alpha particles
Since atoms are electrically neutral, the demonstration that negatively charged electrons are present in matter implied that positively charged species must also be present. Scientists were however unsure as to how these were arranged. Thomson proposed a model in which an atom consisted of a positively charged sphere of matter in which the electrons were embedded. But Ernest Rutherford would go on to carry out an experiment disproving this model. Rutherford fired alpha particles (positively charged particles emitted from a radioactive source) at very thin gold foil. If Thomson’s model were correct, all the alpha particles should have smashed through the gold foil, a little like a bullet passing through paper. To Rutherford’s surprise, whilst the majority of alpha particles did pass through the foil with only minor deflections, a small number (about 1 in 20000) were deflected by angles greater than 90°. Rutherford described it as “quite the most incredible event that has ever happened to me in my life. It was almost as incredible as if you fired a 15-inch shell at a piece of tissue paper and it came back and hit you.” Rutherford set about trying to interpret the results and in 1911, two years after his famous experiment, he came up with an explanation. Because like charges repel, Rutherford reasoned that the alpha particles could only be reflected back if they encountered a dense region of positive charge. The tiny number of deviations thus suggested an atomic structure consisting of a very dense centre of positive charge, the nucleus, surrounded by a large volume of mainly empty space containing the electrons, a model consistent with the observation that most of the alpha particles passed directly through the foil.
A simulation of Rutherford’s gold foil experiment
Rutherford bombarded different elements with alpha particles and found that the nuclei of boron, nitrogen, fluorine, sodium, aluminium and phosphorus all gave out the same positively charged particle which he named the proton. In 1932 James Chadwick bombarded beryllium with alpha particles, producing a highly penetrating stream of particles. He found that they were not deflected by electric or magnetic fields, showing that they were not electrically charged. Chadwick named his particle the neutron. Rutherford and Chadwick had discovered the components of atomic nuclei. Further work by physicists would show that the proton and neutron are not fundamental particles and are in fact made up of quarks, elementary particles which can never be found in isolation, but this is beyond the scope of this article. Instead we will focus on the fascinating world of electrons in atoms.
The nature of light
With strong evidence in support of a positively charged nucleus, Niels Bohr used spectroscopy (the study of matter using electromagnetic radiation) in order to gain insight into the arrangement of the electrons in an atom. In order to comprehend Bohr’s work, we need to understand the nature of electromagnetic radiation. Electromagnetic radiation is a form of energy consisting of oscillating electric and magnetic fields.

Electromagnetic radiation as a wave (creative commons)
These oscillations travel through space at a speed, c, which is equal to 2.998 x 108 m s-1, known as the speed of light. Visible light is a form of electromagnetic radiation as are radio waves, microwaves and X-rays. These types of radiation each have a characteristic wavelength, λ.The wavelength is related to frequency, f, by the following equation:
c (m s-1) = λ (m) f (s-1)
After studying the emission of light from hot objects, Max Plank proposed the idea that electromagnetic radiation could only be emitted or absorbed in packets or quanta of radiation, which were later called photons. He proposed that the energy, E, of a photon is proportional to the frequency of the radiation (equal to a constant, h, multiplied by the frequency) where h is plank’s constant and has a value of 6.626 x 10-34 J s.
E (J) = h (J s) f (s-1)
Plank’s theory suggests that matter is only able to emit and absorb energy in whole number multiples of hf such as hf (1 photon), 2hf (2 photons) and 3hf (3 photons), etc.
Einstein recognised the ability of Plank’s theory to explain an experimental observation known as the photoelectric effect. When ultraviolet radiation strikes a metal surface, electrons are ejected. Electrons are only ejected when the frequency of the ultraviolet radiation is above a certain threshold, specific to the metal. Once the threshold frequency is passed electrons are ejected, regardless of the intensity of the radiation. Einstein realised that instead of behaving like a wave, the UV light behaves as a stream of tiny particles (photons). He reasoned that electrons can only be ejected if the incoming photons transfer a minimum value of energy to atoms on the metal surface. If a photon does not have enough energy, an electron will not be ejected regardless of the intensity of the radiation (the number of photons that strike the surface per unit time), as none of the individual photons have enough energy.

The photons of the red light do not have enough energy to eject electrons. The green and blue light are both above the threshold frequency for this metal and so the photons can eject electrons from the metal. The photons of the blue light are higher in energy and so the electrons are ejected with more energy. Source: Khan Academy (All Khan Academy content is available for free at www.khanacademy.org)
Einstein’s explanation for the photoelectric effect shows how electromagnetic radiation can be shown to behave as particles. There is however considerable evidence that electromagnetic radiation behaves as a wave. In the early part of the 19th century, Thomas Young demonstrated that when light passes through two closely spaced slits, each slit gives rise to a circular wave (diffraction) which then interfere with each other to give a pattern of dark and light bands.

The dark and light bands seen in Thomas Young’s double slit experiment (creative commons)
Diffraction and interference is typical wave behavior which can also be seen with water waves, as seen in the excellent photograph taken by Tom Holub entitled wave interference patterns (hyperlink to his work below).
Hyperlink to a photo taken by Tom Holub which shows diffraction and interference of water waves
This leaves us with a problem. Is light a wave or does it consist of particles!? Scientists had what may seem a bizarre solution to this problem. Light has both wave-like properties and particle-like properties and thus we must consider it as both a wave and as consisting of particles. This idea is known as wave-particle duality.
 Artist: Unknown, Source: Twitter
Artist: Unknown, Source: Twitter
Spectroscopy and the Bohr model of the atom
So now that we are comfortable with the nature of electromagnetic radiation we can see how Bohr used spectroscopy to further our understanding of the atom. We are all used to the idea that when white light is passed through a prism, the light is separated into its constituent wavelength components resulting in a continuous spectrum.
 Continuous spectrum (creative commons)
Continuous spectrum (creative commons)
Scientists observed that when we pass a high voltage of electricity through gases under reduced pressure, the gases give off light of different colours. This is how modern streetlights function, sodium vapour giving off yellow light. Crucially, when this light is passed through a prism, a line spectrum is observed instead of a continuous spectrum. Niels Bohr came up with a model of the atom which explained this observation.

Line spectrum for calcium (the scale gives the wavelength of light in nanometers). Source: Leibniz Universität Hannover
Bohr proposed that the atom consisted of the positively charged nucleus, suggested by Rutherford, surrounded by electrons orbiting the nucleus rather like the planets orbit the sun. In Bohr’s model, each orbit corresponds to a specific energy. The electrons in orbit further from the nucleus have greater potential energy, much like a tennis ball at the top of a flight of stairs has more potential energy than one at the bottom of the stairs. In his model, only certain electron orbits are allowed and thus only certain electronic energies are allowed. Bohr proposed that when a high voltage of electricity is passed through a gas, the electrons in the atom are excited into higher energy orbits. They do not stay in these high energy levels but fall down to their lower, ground state energy level, much like if we throw a ball up the stairs it falls back down again. Because the first law of thermodynamics states that energy cannot be created or destroyed, the potential energy of the electron in its excited state cannot be destroyed and so when the electron falls back to its ground state the potential energy is converted into another form of energy, light energy. This is similar to how the ball’s potential energy at the top of the stairs is converted into kinetic (movement) energy as it falls down to the bottom of the stairs. Because in Bohr’s model of the atom each orbit corresponds to a specific energy, we only observe certain energies of light (and thus certain wavelengths of light) emitted as the electron falls to its ground state, thus explaining the line spectra.

The Bohr model of the atom. +Ze represents the positively charged nucleus. n = 1 is the lowest energy electron orbit, n = 2 is the next lowest energy orbit etc. The green dot represents an electron. ΔE is the difference in energy between n = 3 and n = 2 released as a photon when the electron falls from its excited state in n = 3 to n = 2. (creative commons)
There are however some important problems with the Bohr model of the atom. Why are only certain orbits of certain energies allowed? And why does the orbiting electron not emit electromagnetic radiation and collapse into the nucleus as predicted by classical physics? Bohr’s model, was an important stepping stone in our understanding of the structure of the atom and the idea of quantised electronic energies is retained in the atomic model used by scientists today. The problems would be resolved in the light of scientist’s further reflection on the nature of matter.
De Broglie’s hypothesis
We have already seen that electromagnetic radiation, which scientists had previously considered a wave, possesses particle like properties. Louis De Broglie suggested that matter may also possess wave properties and that the wavelength, λ, is inversely proportional to the mass, m, as shown in the following equation: λ = h / (mv), where h is Plank’s constant and v is the velocity. According to De Broglie’s hypothesis, everyday objects such as your computer and books have wave properties, but the tiny value of Plank’s constant (6.626 x 10-34 J s) mean that these objects have wavelengths so small that we can’t see their wave properties. Crucially, as the mass of the object decreases, the wavelength increases. Millikan calculated the mass of the electron as 9.10 x 10-28 g and evidence suggests that it is reasonable to consider the speed of an electron in an atom (using the Bohr model) as approximately a tenth of the speed of light, 2.998 x 108 m s-1. In order to calculate the wavelength of the electron, we need to use the De Broglie equation, λ = h / (mv):
6.626 x 10-34 kg m2s-1 / [(9.10 x 10-31 kg) x (0.1 x 2.998 x 108 m s-1)] = 2.46 x 10-11 m
According to De Broglie’s equation, the wavelength of an electron moving at 2.998 x 108 m s-1 is 2.46 x 10-11 m, suggesting the electron exhibits considerable wave behaviour.
C Davisson and G.P. Thomson: Diffraction of the electron
De Broglie’s hypothesis was proved right in 1925 when, in independent experiments by G.P. Thomson and Clinton Davisson, the wave properties of electrons were observed for the first time. They found that as electrons passed through a crystal, they were diffracted. This showed that the moving electrons in the crystal exhibit the same kind of wave properties as electromagnetic radiation.

Electron diffraction pattern. Source: Lambda scientific systems
The story of two Nobel prizes
The bizarre nature of the quantum world (the world smaller than the atom) is summed up by the work of father and son scientists J.J. Thomson and G.P. Thomson. The Nobel prize in physics was awarded to J.J. Thomson in 1906 for his work showing that electrons are particles. G.P. Thomson was awarded the Nobel prize in 1937 for showing that electrons are waves. Both of them were right!
Why do electrons have quantized energy?
We said that Niels Bohr could not explain why only certain energies were allowed for the electron in his model of the atom. Indeed, it’s not obvious why the energy of a particle should be quantized, but it makes sense if we think of an electron as a wave. Think of a guitar string. If you make it vibrate, it can vibrate as below:

Standing wave. First harmonic.
However, it could also vibrate as such:

Standing wave. Second harmonic with a single node in the middle (and two nodes where the string is fixed).
Third and fourth possible vibrations are shown below:

Standing wave. Third and fourth harmonic with two and three nodes respectively (plus the two nodes where the string is fixed).
The vibrating string has to vibrate with the frequency taking on only certain values because the wavelength has to be an exact divisor of the length of the string. Because the frequency is proportional to energy, E (J) = h (J s) f (s-1), the vibrating string has quantized energy levels.
Now that we know that an electron can behave as a wave, if we think of an electron orbiting the nucleus as a string looped back on itself we can see how, just as with our guitar string, only certain wavelengths are possible and each wavelength has an associated frequency and energy!

An electron in orbit presented as a standing wave. Source: Analytical spectroscopy
Usain Bolt and the Heisenberg uncertainty principle
Usain Bolt set the 100 m world record in 2009. Using classical physics, we were able to measure his time, 9.58 s, his average velocity, 10.4 m s-1, and taking his mass as 94 kg, his average momentum, 978 kg m s-1. We could have measured his location, velocity and momentum at any given time with great accuracy. Can we do the same with an electron? We have seen that the electron has significant wave properties. The problem with waves is that they extend in space. How then can we measure their location? Heisenberg proposed that we could not measure the position and momentum of an electron at any given time. In order to understand his reasoning, think about how we located Usain Bolt. We detected light that bounced off him with our eyes. The photons of light did little to alter his position or momentum so we can know both his position and momentum at the same time. However, due to the tiny mass of the electron, the photons needed to detect it give it a little kick which changes its motion in an unpredictable way. In order to reduce the magnitude of this kick we could use photons of longer wavelength and thus lower energy giving us a better idea of the electron’s momentum. Unfortunately, by using photons of longer wavelength, the accuracy of our measurement of the electron’s position is reduced. Heisenberg therefore concluded that there is an uncertainty in simultaneously knowing the position and momentum of an electron because the more accurately one is known the less accurately the other is known.
Schrödinger and the wavefunction
The Heisenberg uncertainty principle shows us that electrons can never be precisely located in space. Schrödinger proposed a solution to this problem in which he incorporated both the wavelike and particle like properties of the electron. He first treated the electron as a standing wave, much like the guitar string with its allowed quantized energies. By solving the Schrödinger equation, which requires advanced mathematics, he obtained a series of mathematical functions called wavefunctions. The wavefunction squared gives a smeared out picture of the electron’s habits, a bit like blurred pictures of the vibrating guitar string, except in 3D. These are known as atomic orbitals and represent the probability of finding an electron at this location. Each orbital can contain a maximum of two electrons.
The lowest energy atomic orbital is spherical and is known to scientists as the 1s atomic orbital.

An illustration of the shape of the 1s orbital. Source: Nick Greeves
The 2s atomic orbital is also a sphere but it is higher in energy and like the first harmonic for the guitar string contains a node. For atomic orbitals, the node represents a point where the electron can never be found.

An illustration of the shape of the 2s orbital. Source: Nick Greeves
The 2p orbital also has one node but the orbital’s shape is not spherical and contains a planar node. The 2p orbital is directional (it points along an axis) and there are three 2p orbitals of the same energy which we call 2px, 2py and 2pz. The planar node of the three 2p orbitals gives them just slightly more energy than a 2s orbital, with its spherical node.
 An illustration of the shape of the three 2p orbitals. Source: Nick Greeves
An illustration of the shape of the three 2p orbitals. Source: Nick Greeves
There are many more orbitals which electrons can occupy, the shapes of which are determined by solving Schrodinger’s equation. The atomic orbitals can be thought of as allowed states for an electron, a little like how standing on a step on a staircase is allowed (possible) but standing between the steps is impossible! It should be noted that in the same way that someone doesn’t need to be standing on a step for it to exist, atomic orbitals do not need to have an electron in them!
When trying to picture Schrödinger’s model of the atom, keep in mind that all these atomic orbitals are superimposed on each other. The 1s orbital is not the middle of the 2s orbital, they are separate orbitals in their own rights. The 2s orbital does however occupy some of the same space as the 1s and the 2p orbitals.
A simulation showing how the atomic orbitals are superimposed on each other. No need to watch the full two minutes and 31 seconds!
Experimental evidence in support of Schrödinger’s model of the atom
Feynman once said that “no matter how beautiful your theory, no matter how clever you are or what your name is, if it disagrees with experiment, it is wrong.”
Schrödinger’s model of the electron arrangement of the atom has been found to explain many experimentally observed phenomena in chemistry. It explains how atoms bond to form molecules and the properties of these molecules, such as the magnetic properties of oxygen. By using the geometry of atomic orbitals and molecular orbitals, orbitals found in molecules, scientists can predict and explain the nature of chemical reactions which was impossible using the Bohr model of the atom.

The magnetic properties of liquid oxygen can be explained by a theory of bonding which uses Schrödinger’s atomic orbitals. Source: iusbchemistry
Seeing is believing
The work of scientists has allowed us to probe the structure of the atom even though we cannot see individual atoms with our eyes. Through looking at what led scientists to develop their theories and the evidence they had for them, we have learned about the nature of science. We saw that, before Boltzmann, the majority of the scientific community did not even believe that matter was made of particles, unthinkable today. We saw how scientists once believed that light could only be described as a wave and that electrons could only be described as particles. These striking examples of how science corrects itself exemplify that all scientific knowledge is provisional. The ability of science to correct itself in the wake of new findings is surely its crowning glory.
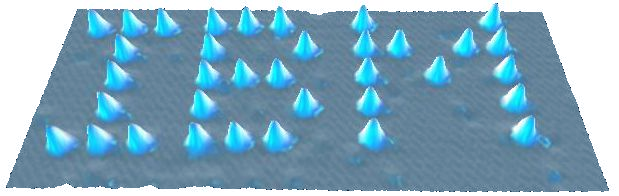
Scientists have now developed the scanning tunnelling microscope (STM), a machine that allows us to create images of surfaces with sufficiently high resolution to detect atoms. The STM constructs an image by analysing the current flowing between the surface of a material under investigation and a probe on the machine. The closer the probe to the surface, the greater the current so by scanning the surface with the probe, a contour map of the surface can be constructed. The image below of xenon atoms on a nickel surface produced by a research team sponsored by IBM is an example of an STM in use. I leave the reader to decide whether this really enables us to “see” individual atoms!
 An STM image of xenon atoms on a nickel surface, showing the electron cloud of the individual atoms of xenon (creative commons)
An STM image of xenon atoms on a nickel surface, showing the electron cloud of the individual atoms of xenon (creative commons)